Use the calculator below to work out the Voltage, Current or Resistance based on the known values. Where Current = Voltage / Resistance.

Voltage:			V
Current:			Amps
Resistance:			Ω

 

Ohms Law Explanation

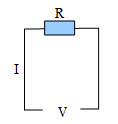1) A basic electrical circuit has three components

Voltage (V)
Amperes (A)
Resistance (Ω)

2) Ohms law states that these 3 components are always related in the same way mathematically

They are related as follows

V = I x R (Volts = Amps x Resistance)
I = V / R (Amps = Volts / Resistance)
R = V / I (Resistance = Volts / Amps)

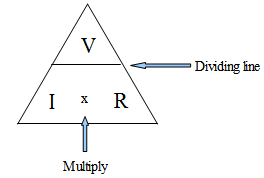
The above triangle is the easiest way to remember this relationship. If you cover with your finger the figure you want to work out, what’s left is the sum you need to do. (Note If you cover a letter from the bottom, also cover the x symbol as well.)

• Cover over ‘I’ and you are left with ‘V’ over ‘R’ or the sum V / R
• Cover over ‘V’ and you are left with ‘I’ x ‘R’
• Cover over R and you are left with V / I

Now if we replace the letters with numbers we can calculate the number for the part of the circuit that is missing. Here are some worked examples.

If you want to know V and you know I = 4 and R = 2 here’s the sum

V = I x R
V = 4 x 2
V= 8

To know R when V = 8 and I = 4 here’s the sum

R= V / I
R = 8 / 4
R = 2

Resistance (R):			Ω
Inductive Reactance (XL):			Ω
Capacitive Reactance (XC):			Ω

We calculate Total Impedance using the formula

 

Where

• Z = Total Impedance measured in Ohms (Ω)
• R = Resistance measured in Ohms (Ω)
• XL = Inductive Reactance measured in Ohms (Ω)
• XC = Capacitive Reactance measured in Ohms (Ω)

In order to practice calculating Total Circuit Impedance (Z) we can use an example circuit with a Resistor, Inductor and Capacitor in series. See Fig 1.

Fig 1.

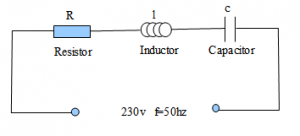
We measure

• R = Resistance measured in Ohms
• l = Inductance measured in Henry’s
• c = Capacitance measured in Farads
• f = Frequency measured in Hertz

We are given R so this figure can go into the formula for Total Impedance (Z) with no further calculation required.

However we are only given inductance (l) and so we need the following formula to calculate Inductive Reactance (XL)

XL = 2πfl

Note – inductance (l) can often be given in milli-Henry’s (mF) or micro-Henry’s (μH)

When inductance (l) is give in milli-Henry’s (mH) the figure must be multiplied by 10ˉ3

When inductance (l) is given in micro-Henry’s (μH) the figure must be multiplied by 10ˉ6

Similarly we are only given capacitance (c) and so we need the following formula to calculate Capacitive Reactance (XC)

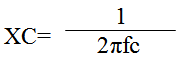
Note – Capacitance (c) can often be given in milli-Farads (mF) or micro-Farads (μF)

When capacitance (c) is give in milli-Farads (mF) the figure must be multiplied by 10ˉ3

When capacitance (c) is given in micro-Farads (μF) the figure must be multiplied by 10ˉ6

Worked example

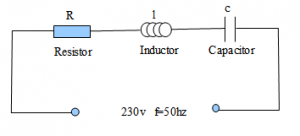
• R = 20 Ohms (Ω)
• l = 40mH
• c = 20 μH
• f = 50hz

We can calculate Inductive Reactance as follows.

We can calculate Capacitive Reactance as follows.

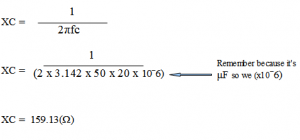
We now have all we need to calulate Total Circuit Impedance (Z)

• R = 20(Ω)
• XC = 159.13(Ω)
• XL = 12.568(Ω)

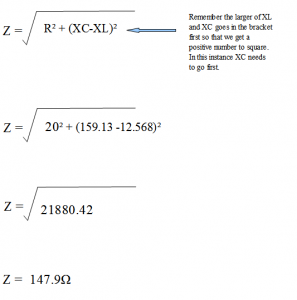
Now try it on your own making up you own values for f (frequency), l (inductance), c (capacitance), and R (resistance).